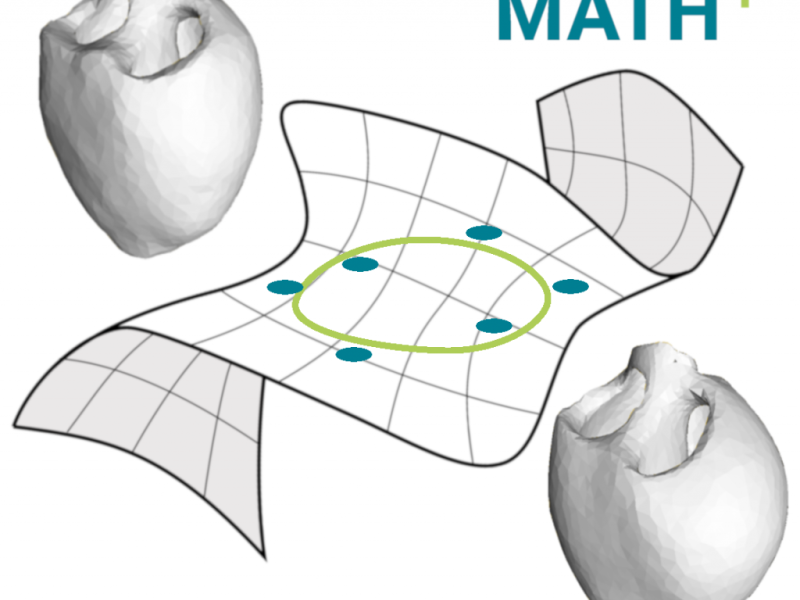
The aim of the project is the development of novel methods for the identification of systematic differences in empirically defined distributions of shape trajectories. The focus will be on the derivation of hierarchical statistical models that account for the correlation inherent to time series of shapes. To this end, we will generalize the mixed-effects framework to manifold-valued data based on Riemannian spline models. The development will be driven by applications from cardiology and archaeology. In particular, a better understanding and algorithmic treatment of shape trajectories will support the diagnosis and therapy of heart diseases and will establish a quantitative method for the dating of ornaments and thereby archaeological buildings.