The impact of mechanical loading of articular cartilage on the onset and progression of osteoarthritis is well known. The actual mechanisms of this connection however, remain unclear. One main reason is that in-vivo measurement of the stress and strain distribution within the cartilage during motion are not possible.
This project aims at estimating stress distributions in knee cartilage resulting from common body motions. Our approach relies on the coupling of a biomechanical model of the lower limb dynamics to a finite element model of the knee for computing contact mechanics. An inverse problem ansatz allows for the estimation of local forces and resulting stresses based on medical gait data. This leads to a large optimization problem with non-smooth contact constraint. We aim at developing efficient optimization algorithms that are capable of dealing with such heterogeneous models in the presence of non-smootheness. In particular, we combine augmented Lagrangian composite step methods with a nonlinear semi-geometric multigrid contact solver.
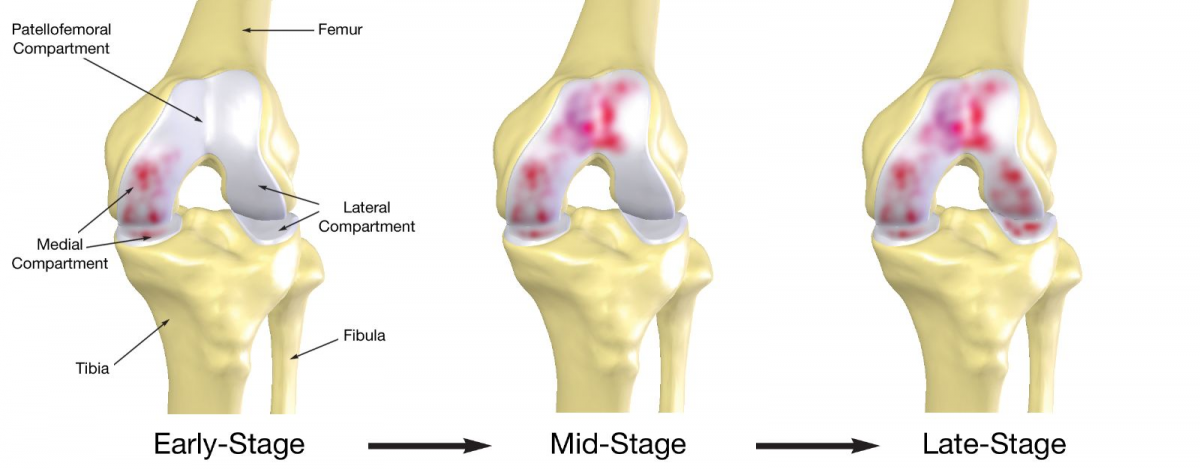
Typical progession of osteoarthritis in the knee
Dynamical Multibody Model
The multibody model describes the position and orientation of the muscoskeletal system during motion. The bones are modelled as rigid bodies connected by mechanical joints, while muscles, tendons and ligaments are taken into account as forces and torques acting on the bones. Additional constraints on the motion, such as friction and ground contact can be considered. The equations of motion for the system result from the conservation of momentum - Newton's second law, which relates the velocity of the bones to the acting muscle forces. Multibody dynamics typically leads to differential algebraic equations (DAEs), which require a careful choice of consistent inital data and numerical integration schemes.
The available medical gait data consists of skin marker positions such as reaction forces due to contact with the ground, both as time series during a full gait cycle. The estimation of the local stress distribution is based on this data.
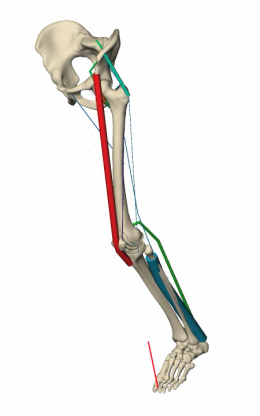
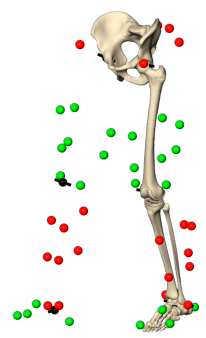
Multibody model including bones, muscles and tendonds such as skin marker positions
Contact Model
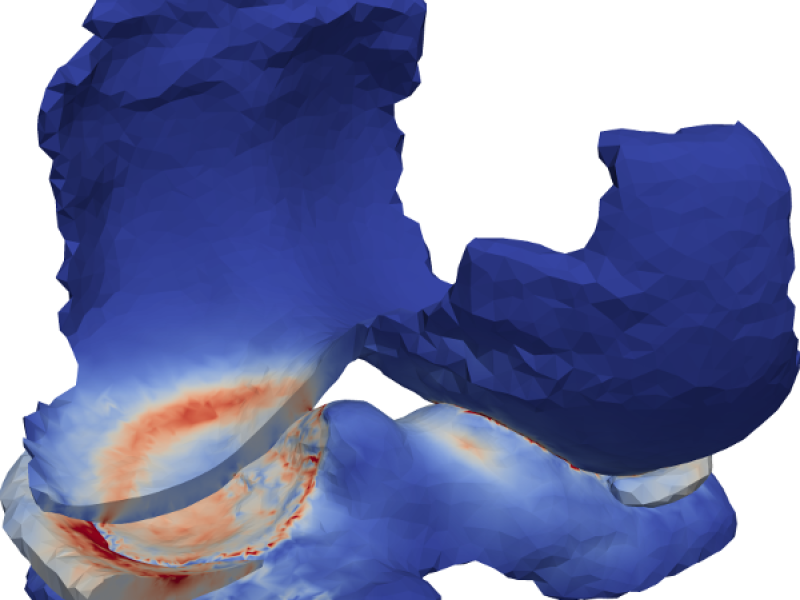
For the computation of contact mechanics in the knee cartilage a three- dimensional mesh of the geometry and all relevant structures in needed. It is obtained by a magnetic resonance imaging (MRI) scan of the knee and a subsequent segmentation, which allows for resolution of patient specific anatomy features.
The resulting mesh is the base for the contact model in the knee joint. Enforcing non-penetration of the bodies leads to a variational inequality, which introduces theoretical and numerical difficulties. One the one hand, the non-penetration condition introduces non-smoothness into the model. On the other hand, the discretization of this condition must be tackled by solvers for inequality constrained optimization problems.
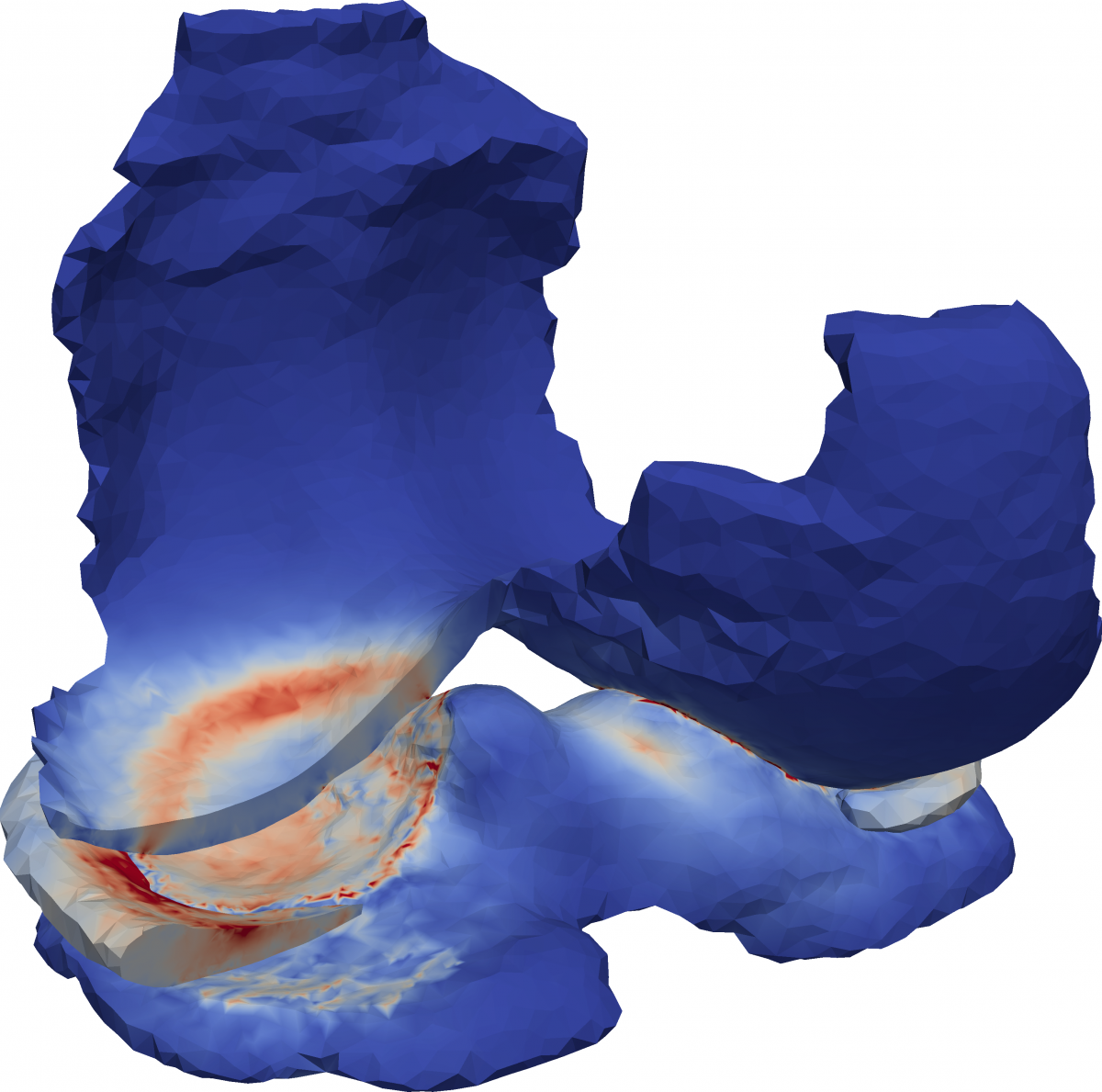
Patient specific knee geometry
Identification of stress
For an estimation of local stresses, we need to embed both models into a complete description of the system behavior. This leads to a large heterogeneous model with contact constraints. A Bayesian ansatz for the identification results in an optimal control problem for this model. In particular the non-smoothness due to the contact poses a challenge in this optimization problem.