Neurotransmission denotes the passing of stimuli from a neural cell to a target cell at specific junction points called synapses. The aim of this project is to increase our understanding of the underlying processes via spatio-temporal deterministic and stochastic modelling.
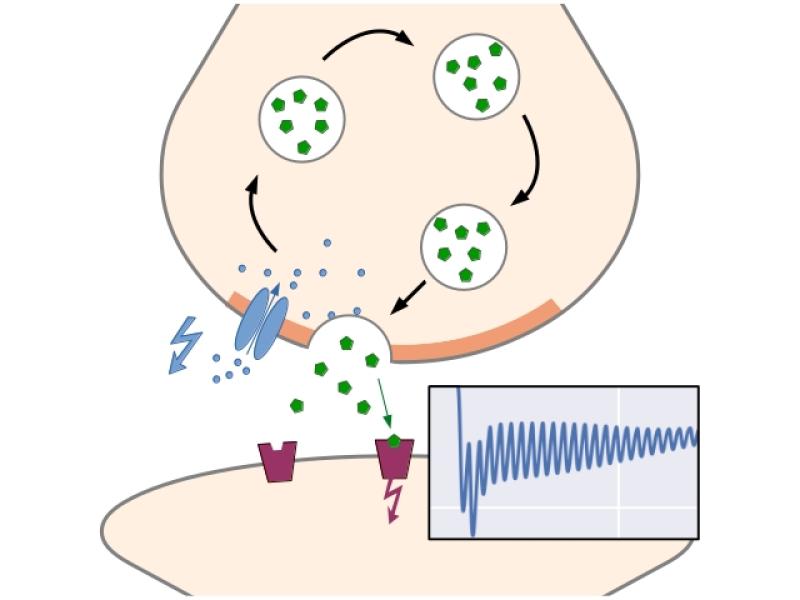
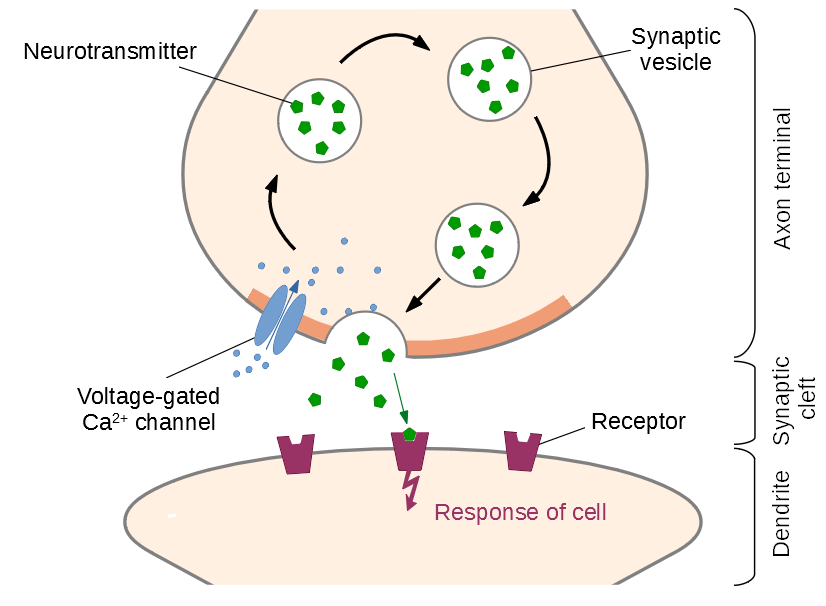Figure 1: Basic function of a chemcial synapse: Upon arrival of an action potential, voltage-gated calcium channels open in the active zone. The inflowing Ca2+-ions bind to proteins on the surface of primed (docked and prepared) vesicles, causing them to release neurotransmitters into the synaptic cleft. After diffusing across the cleft, the molecules activate receptors in the postsynaptic membrane, triggering a new action potential.
Despite — or rather, because! – of its unreliable and plastic nature, synaptic function is a key player in almost all neural activity and suspected to be of great importance especially for learning processes in the brain. This makes it a very attractive area of research not only for neuroscientists, but also physicists, mathematicians and information scientists. Since the size scale of vesicles as well as the synaptic cleft is in the order of few nanometers, well below the diffraction limit, dynamic and precise imaging of neurotransmission processes is currently very difficult. We can however measure the excitatory junction currents resulting from many synaptic contact points in the postsynaptic cell using voltage- or patch-clamp method. In order to gain information from these currents, mathematical modelling is needed.
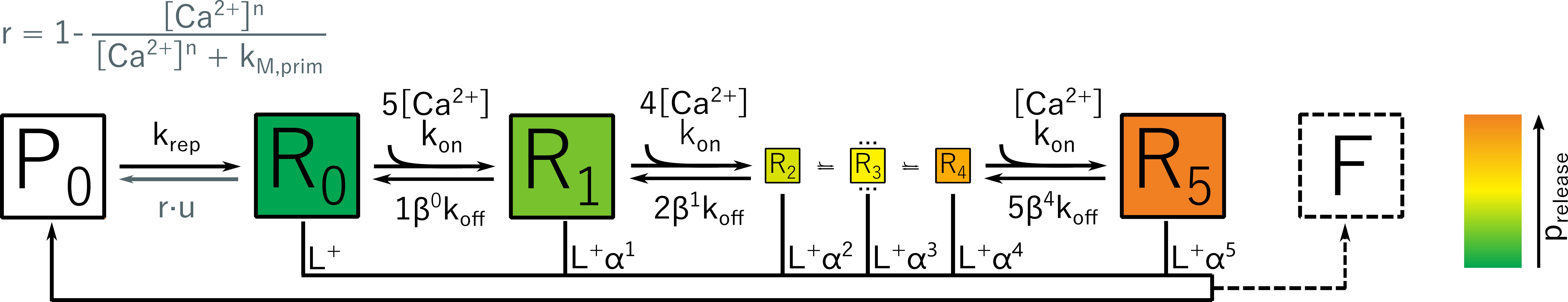
Figure 2: Unpriming model by Kobbersmed et al.: Vesicles are primed for fusion at rate krep, and the binding of calcium ions increases the fusion rate. Up to five ions can be bound. The vesicle can also become unprimed again at a rate that depends on the local calcium concentration.
Based on the unpriming model by Kobbersmed et al, we have developed a method for direct computation of the exact first- and second-order moments for models that generate the postsynaptic current by convolving an impulse response function with the output signal of a linear reaction network [Ernst et al., 2022]. This renders expensive stochastic simulations obsolete. We also investigated if a model extension could help explore the rate-limiting factor during sustained synaptic activity [Ernst et al., 2023], which is currently an unsolved problem in biology.
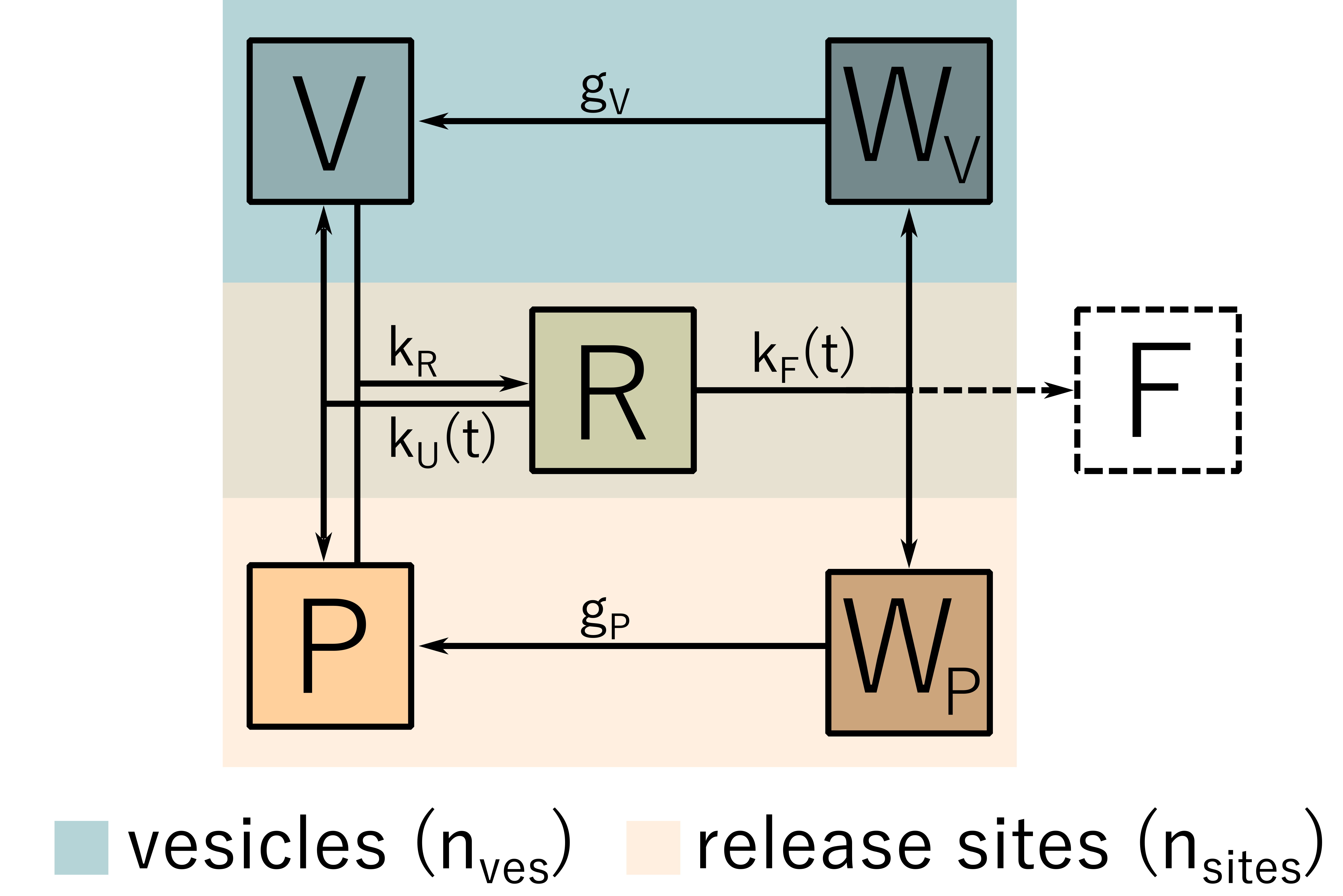
Figure 3: Recovery model. A freely available vesicles V attaches to a release site P. From the resulting joint state R, fusion occurs, transferring both the vesicle and the release site into their recovery states WV and WP, respectively.
References:
J. R. Kobbersmed, A. T. Grasskamp, M. Jusyte, M. A. Böhme, S. Ditlevsen, J. B. Sørensen, and A. M. Walter. Rapid regulation of vesicle priming explains synaptic facilitation despite heterogeneous vesicle: Ca2+ channel distances. eLife 9 (2020): e51032. https://doi.org/10.7554/eLife.51032.
